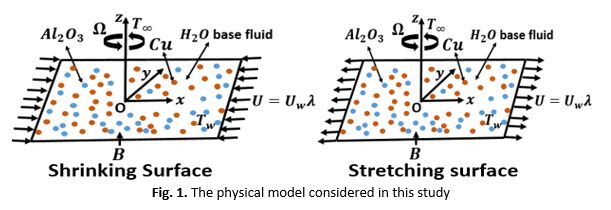
Three Dimensional MHD Hybrid Nanofluid Flow with Rotating Stretching/Shrinking Sheet and Joule Heating

Abstract

A three-dimensional hybrid nanofluid flow over a stretching/shrinking sheet is numerically studied. The hybrid nanofluid being considered in this study used water as the base fluid and mixed with two types of solid nanoparticles, namely alumina (Al₂O₃) and copper (Cu). The main focus of the current study is to examine the effect of magnetic field, Joule heating, and rotating sheet on the velocity,  and temperature profiles. In addition, the impact of suction and stretching sheet on the variations of reduced skin friction, , and reduced heat transfer are studied as well. The fluid flow and heat transfer problem presented in this study is governed by a system of nonlinear partial differential equations (PDEs), which is then transformed into the corresponding system of high order nonlinear ordinary differential equations (ODEs) using similarity variables. The resulting system of higher order nonlinear ODEs is solved numerically using a boundary value solver known as bvp4c, which operates on the MATLAB computational platform. Results revealed that dual solutions exist for shrinking sheet while unique solutions are observed for stretching sheet with various values of Cu nanoparticles volume fraction and magnetic parameter. Dual solutions also exist for the value of the suction parameter greater than its critical point with various values of Cu nanoparticles volume fraction. Velocity profile of the hybrid nanofluid increases alongside with the value of magnetic parameter but declination was observed in the profile of  and temperature, for both solutions as the value of Cu nanoparticles volume fraction increases. When the value of rotational parameter increases, both velocity and  profiles increase for both solutions. This indicates that the momentum boundary layer thickness increases with increasing values of  for both solutions, but thermal boundary layer thickness decreases for the first solution and increases for the second solution. Finally, an increment in the value of Eckert number causes the temperature of the hybrid nanofluid to rise as well for both first and second solutions.